At the end of last month, we had a preliminary layout of the configuration of our airplane and had made an initial estimate of its takeoff gross weight. We will now move on to sizing the most aerodynamically important component of the airplane: the wing.
The size of an airplane’s wing is determined by many factors from different parts of the flight envelope. The designer must consider takeoff and landing distance, stall speed, climb performance, ceiling, and cruise performance. Over the next few months, we will consider all of these, but for this month we will start with cruise performance.
Even though other constraints might force the wing to be bigger than optimum for cruise, it’s useful to start out with a cruise-optimized wing and then compromise as necessary to meet other requirements.
The drag of a lifting wing is comprised of two components: parasite drag and induced drag. Induced drag is the drag caused by the production of lift and is a function of the lift and the wingspan squared. Parasite drag is the drag caused primarily by skin friction of the air scrubbing over the surface and is a function of the wetted area and shape of the airfoil.
If we look at this in terms of non-dimensional coefficients, we find that the parasite drag coefficient (Cd0) is constant, and the induced drag coefficient varies with lift coefficient squared:
Cdi=CL2/(π e AR)
Where e is the span efficiency of the wing and AR is the wing aspect ratio.
Optimum Lift Coefficient
For any given wing planform and airfoil, the L/D (lift to drag ratio) of the wing varies as a function of lift coefficient. Figure 1 shows the variation in L/D for an example wing. In this particular case, the wing achieves its best L/D at a CL of approximately 0.4. If the lift coefficient is higher or lower than this, the L/D of the wing will be lower. Accordingly, if the designer has chosen this basic wing design, it should be sized so it is flying at this lift coefficient at cruise.
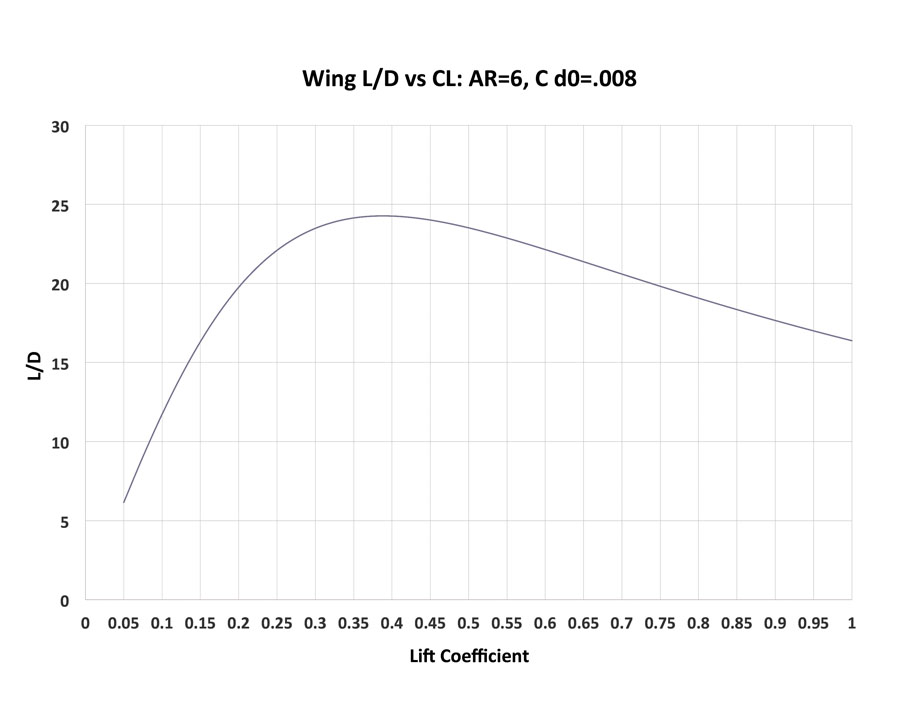
Figure 1: Variation in L/D for an example wing. In this particular case, the wing achieves its best L/D at a CL of approximately 0.4.
It’s possible to calculate this optimum wing lift coefficient directly. Classical wing theory tells us that maximum L/D is achieved when exactly 1/2 the drag of the wing is induced drag and 1/2 is parasite drag (Cdi= Cd0). From this it is possible (with more algebra than I will include here) to determine that for any given planform and airfoil: Optimum lift coefficient is given by:
CLopt=sqrt(π e AR Cd0)
Wing Size
Once we have chosen the planform and airfoil of the wing, and know the optimum CL the wing should operate at in cruise to minimize drag, we need to size the wing properly so it is operating at CLopt at the airplane’s cruise condition.
The lift coefficient the wing flies at in 1-G steady-state flight is a function of the wing loading, the density of the air (altitude), and airspeed.
For any given altitude and airspeed, level-flight lift coefficient is a function of airspeed:
CL = 2W/(S ρ V2)
For this equation, W is the gross weight in pounds, S is the wing area in square feet, V is airspeed in feet per second, and ρ is the density of the air in slugs/ft3. (A slug is the standard unit of mass in the English system of units. It’s not necessary to know more than this since the air density in appropriate units is available from a standard atmosphere table.)
It’s possible to invert this equation to solve for the wing loading for a given lift coefficient, so for our optimum wing:
(W/S) = 1/2 ρ V2 CLopt
This equation gives us the optimum wing loading for our wing for the given airspeed, weight, and altitude. Using this and the actual gross weight, we can calculate the optimum wing area.

Figure 2: Optimum wing loading for an airplane intended to fly at 150 knots. Two curves are shown, one for an AR=6 wing and one for an AR=12 wing, both with the same airfoil.
Figure 2 shows the results of such a calculation for an example airplane intended to fly at 150 knots true airspeed. Two curves are shown, one for an AR=6 wing and one for an AR=12 wing, both with the same airfoil.
Notice first, that for any altitude, the higher aspect ratio wing has a higher optimum wing loading. It will also have a higher L/D, but because of the higher optimum wing loading, it is more likely to be constrained by other considerations like stall speed or takeoff and landing performance. If these constrain wing loading, we might not be able to get the full aerodynamic benefit of increasing aspect ratio because the wing will be forced to be bigger than optimum. The higher aspect ratio wing will also tend to be heavier. Space does not permit a detailed exploration of these trade-offs this month, but we can see that ahead lies an interesting exercise trading off aspect ratio, wing weight, and aerodynamic requirements to get to a final wing design.
The second, and more important, thing to note is that the optimum wing loading drops steadily with increasing cruise altitude. It is critical for a designer to understand this. A very common error in the design of experimental light planes is to do all of the performance calculations using sea-level standard-day atmospheric properties.
Sea-level standard-day conditions (zero density altitude) are never actually encountered in cruise flight unless you are flying over Death Valley in the winter. Using zero density altitude for wing sizing will inevitably result in an under-winged airplane.
Looking at Figure 2, we can see that at sea level, the optimum wing loading for our AR=6 wing is about 30 pounds per square foot. For a density altitude of 9000 feet, which is the altitude that a normally aspirated engine delivers 75% power at full throttle, the optimum wing loading is about 22 pounds per square foot. This means that the optimum wing for the realistic cross-country cruise point is 36% larger than the wing that is optimized at sea level.
The consequences of using the smaller “sea level” wing can be considerable. The first is that the airplane will actually be slower than the airplane with the larger properly sized wing when flying at its real cruise altitude.
The smaller wing will also be a poorer performer in other areas. Because of the higher wing loading, stall speed will increase by 17%. The airplane will use more runway to take off and land, and will also have a poorer rate of climb and ceiling. In general, it’s usually better to err on the side of making the wing a bit larger rather than too small.
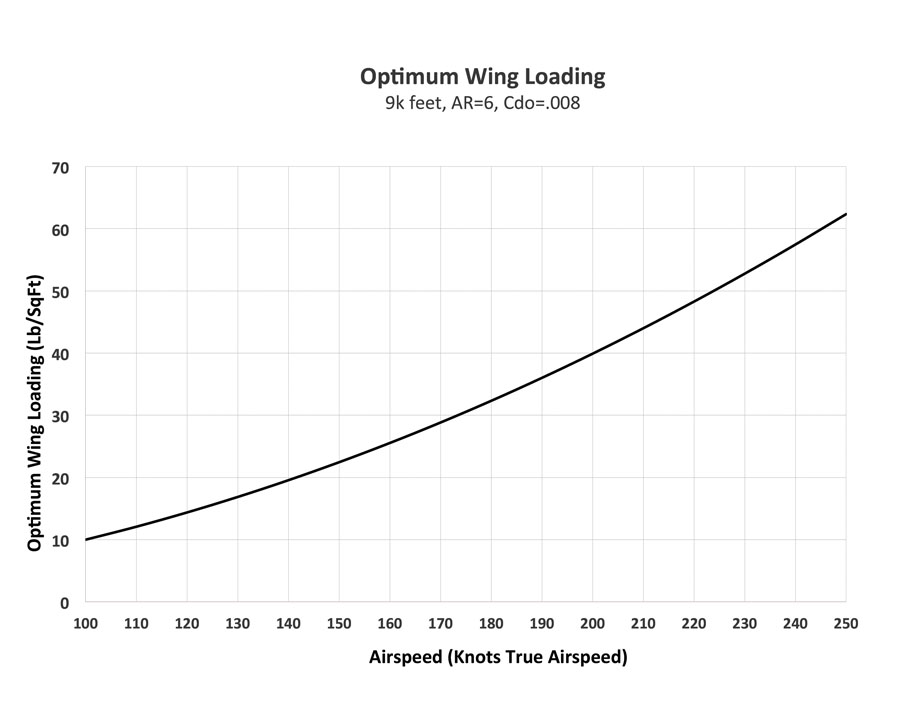
Figure 3: Optimum wing loading for an AR=6 wing at 9000 feet density altitude. Notice that the optimum wing loading increases with increasing speed.
Figure 3 shows the variation of optimum wing loading with true airspeed at 9000 feet density altitude for our AR=6 wing. Notice that the optimum wing loading increases with increasing speed. Once again, the designer should take care to optimize the wing at the desired cruise speed.
Trying to optimize the wing for maximum speed, rather than cruise speed, will result in an airplane with a too-small wing that might be slightly faster with 100% power, but will actually be slightly slower at cruise power. It will also have the higher stall speed, longer takeoff and landing distance, and poorer rate of climb that come with a smaller wing.
It’s tempting to try to get the highest maximum speed possible for the marketing brochure, but the airplane with the cruise-optimized wing will be the better machine for the way it is actually flown in real-world operations.

![]()
Barnaby Wainfan is a principal aerodynamics engineer for Northrop Grumman’s Advanced Design organization. A private pilot with single engine and glider ratings, Barnaby has been involved in the design of unconventional airplanes including canards, joined wings, flying wings and some too strange to fall into any known category.